Lesson 3 introducing polynomials answer key – Welcome to Lesson 3: Introducing Polynomials Answer Key, the ultimate guide to unraveling the intricacies of polynomials. This comprehensive resource provides clear explanations, engaging examples, and expert guidance, empowering you to conquer polynomial operations, functions, equations, and identities with confidence.
Prepare to delve into the fascinating world of polynomials, where numbers and variables intertwine to form powerful mathematical expressions. With our expert guidance, you’ll master the art of polynomial manipulation, gaining a deep understanding of their behavior and applications.
Lesson 3: Introducing Polynomials: Lesson 3 Introducing Polynomials Answer Key

Polynomials are algebraic expressions that consist of variables and coefficients. They are widely used in mathematics, science, and engineering to model various phenomena and solve complex problems.
Polynomial Basics
A polynomial is an expression of the form:
P(x) = anx n+ a n-1x n-1+ … + a 1x + a 0
where a n, a n-1, …, a 1, a 0are constants, and x is the variable.
The degree of a polynomial is the highest exponent of the variable. Polynomials can be classified into different types based on their degree:
- Linear polynomial: Degree 1
- Quadratic polynomial: Degree 2
- Cubic polynomial: Degree 3
- Quartic polynomial: Degree 4
- Quintic polynomial: Degree 5
Polynomial Operations, Lesson 3 introducing polynomials answer key
Polynomials can be added, subtracted, multiplied, and divided using specific rules:
Addition and Subtraction
To add or subtract polynomials, simply combine like terms:
(anx n+ a n-1x n-1+ … + a 1x + a 0) ± (b nx n+ b n-1x n-1+ … + b 1x + b 0) = (a n± b n)x n+ (a n-1± b n-1)x n-1+ … + (a 1± b 1)x + (a 0± b 0)
Multiplication
To multiply polynomials, use the distributive property:
(anx n+ a n-1x n-1+ … + a 1x + a 0) × (b mx m+ b m-1x m-1+ … + b 1x + b 0) = c n+mx n+m+ c n+m-1x n+m-1+ … + c 1x + c 0
where c i= a 0b i+ a 1b i-1+ … + a ib 0
Division
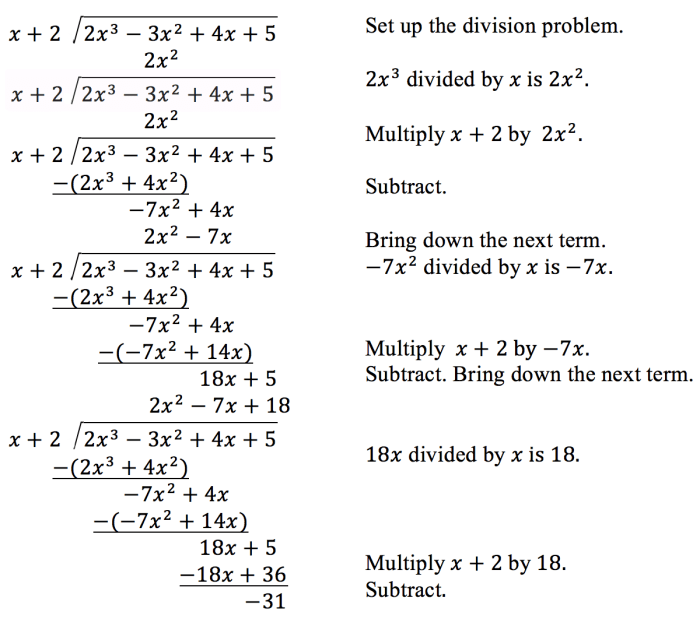
Polynomials can be divided using long division or synthetic division:
- Long division:Similar to long division for numbers, except with polynomials.
- Synthetic division:A shortcut method for dividing polynomials by a linear factor (x – a).
FAQ Explained
What are the different types of polynomial coefficients?
Polynomial coefficients can be positive, negative, or zero, and they determine the behavior of the polynomial.
How do I solve polynomial equations of different degrees?
There are various methods for solving polynomial equations, including factoring, completing the square, and using the quadratic formula.
What are the key features of polynomial functions?
Polynomial functions are characterized by their degree, zeros, extrema, and end behavior.