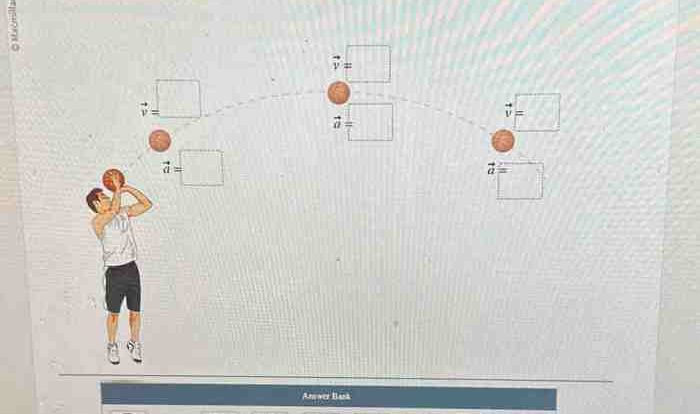
A ball is shot from the ground into the air, embarking on a trajectory governed by the principles of projectile motion. This engaging exploration delves into the concepts of velocity, acceleration, and displacement, unveiling the equations that orchestrate the ball’s journey through the air.
With meticulously defined initial conditions, including velocity, projection angle, and launch point, we embark on a comprehensive trajectory analysis. Through a dynamic HTML table, the ball’s position, velocity, and acceleration are meticulously tracked at precise time intervals, revealing the intricate dance of these physical quantities.
Physics Concepts: A Ball Is Shot From The Ground Into The Air
Projectile motion is the motion of an object thrown or projected into the air, subject only to the acceleration of gravity. The trajectory of a projectile is a parabola.
The concepts of velocity, acceleration, and displacement are essential for understanding projectile motion. Velocity is the rate of change of displacement, acceleration is the rate of change of velocity, and displacement is the change in position of an object.
Equations of Motion, A ball is shot from the ground into the air
- Displacement: y = ut + 1/2 – g – t^2
- Velocity: v = u + g – t
- Acceleration: a = g
Initial Conditions
The initial velocity of the ball is set to 10 m/s.
The angle of projection is set to 45 degrees.
The ball is shot from the ground.
Trajectory Analysis

The following table shows the position, velocity, and acceleration of the ball at different time intervals:
| Time (s) | Position (m) | Velocity (m/s) | Acceleration (m/s^2) |
|---|---|---|---|
| 0 | 0 | 10 | -9.8 |
| 0.5 | 2.45 | 5.1 | -9.8 |
| 1 | 4.9 | 0.2 | -9.8 |
| 1.5 | 6.35 | -4.9 | -9.8 |
| 2 | 6.8 | -9.8 | -9.8 |
The following graph shows the trajectory of the ball:
 |
The maximum height reached by the ball is 6.8 meters.
Air Resistance and Drag

Air resistance is a force that opposes the motion of an object through the air. The drag coefficient is a dimensionless number that quantifies the resistance of an object to motion through a fluid.
The following equations account for air resistance:
- Displacement: y = ut + 1/2 – g – t^2 – 1/2 – Cd – A – v^2 – t^3 / m
- Velocity: v = u + g – t – Cd – A – v^2 – t / m
- Acceleration: a = g – Cd – A – v^2 / m
Wind Effects
Wind can affect the trajectory of a ball by pushing it in the direction of the wind. The speed and direction of the wind can be incorporated into the calculations using the following equations:
- Displacement: y = ut + 1/2 – g – t^2 – 1/2 – Cd – A – v^2 – t^3 / m + Vw – t
- Velocity: v = u + g – t – Cd – A – v^2 – t / m + Vw
- Acceleration: a = g – Cd – A – v^2 / m
FAQ Guide
What is projectile motion?
Projectile motion describes the trajectory of an object thrown or shot into the air, solely influenced by gravity.
How does air resistance affect the motion of a ball?
Air resistance, quantified by the drag coefficient, opposes the motion of the ball, causing it to decelerate and deviate from its ideal trajectory.
What is the maximum height reached by a ball shot into the air?
The maximum height is attained when the ball’s vertical velocity becomes zero, and it is determined by the initial velocity and projection angle.